Poker Odds Chart Explained
Poker Probabilities Introduction Five to Nine Card Stud. The following tables show the number of combinations and probability for each poker hand using the best five cards from out of 5 to 10 cards.
- Push Fold Chart: Nash Poker Chart (Poker Shove Chart) The purpose of this comprehensive article is to serve as the only resource you’ll ever need to use in relation to the use of charts in poker. Consisting of contents ranging from bankroll suggestions to poker preflop charts to pot odds charts, this incredible resource has it all!
- Rough Odds for Texas Hold’em. Playing poker is about playing the odds. The following list gives the odds for outcomes in Texas Hold’em hands. When you realize how heavily the odds are stacked against you, you may want to rethink going all-in before the flop with two suited cards. Use the odds to your advantage.
- Poker odds charts help you to determine how much you can call when trying to complete a drawing hand, such as a flush or a straight draw. By comparing your pot odds with the odds of completing your draw, you can work out whether or not it will be profitable to call certain sized bets from your opponents.
- With 15 outs, 4 x 15 = 55 percent you'll complete that straight or flush with the next two draws. However, when you are calculating the odds that a single draw will improve your hand, you multiply the outs by two rather than 4. With 15 outs, 2 x 15 = 30 percent chance.
Poker Odds Chart Explained Chart
Pot odds is a fundamental and mathematical concept used in poker. Before you understand what pot odds are, take a look at the options below:
Option A: If you risk 1 cookie, you will get 2 cookies.
Option B: If you risk 1 cookie, you will get 5 cookies.
Which option will you choose? Definitely option B. You get 5 cookies for risking 1 cookie!
What if I replace cookie with 1 million dollars? How will it look like?
Option A: If you risk $1 million, you will get $2 million.
Option B: If you risk $1 million, you will get $5 million.
Notice any difference? The values have changed, but the ratio between the reward and risk is still the same. If you have noticed this difference, you have already grasped the meaning of pot odds!
So, without any further ado, let’s delve into the fundamental concept in poker!
What are Pot Odds in Poker?
Poker pot odds are a mathematical expression of risk and reward. Pot odds tell you how much do you need to risk in order to win a reward. It is the ratio between the reward and the risk.
Reward = Pot to be Won
The pot can be at any point of the game – preflop, flop, turn or river.
Risk = How much you need to risk in order to win the pot
The risk is the size of someone’s bet/raise/re-raise you are facing. It is the amount you need to call in order to win the pot.
Pot odds = Reward : Risk
It is the ratio of the size of the pot (reward) to the amount you have to call (risk).
For example:
If there is $6 in the pot and your opponent bets $3, the pot becomes $9. In order to win the pot of $9, you need to call $3. So, what are the odds?
Reward = $9 (Size of the Pot)
Risk = $3 (Size of the Call)
Pot Odds = $9(Reward):$3(Risk)
= 9:3, which can be simplified to 3:1
Hence, the pot odds are 3:1. You can look at these odds in two ways.
- You need to pay 1/3 of the pot for a chance to win the whole pot
- You will get 3X the reward of your risk
Poker Pot Odds Example
Alert! This can be a bit mathematical but an easy hack to learn the odds quickly is available right after the example.
So, have some patience and continue reading…
In this example, we will not be looking at the hole cards so that you are solely focussed on the concept of poker odds.
Here’s the situation: You are on the Button in a $1/$2 6-max game. The Under the Gun (UTG) calls $2. The Middle Position (MP) raises to $5. Let’s calculate the pot odds.
If you don’t know what positions are, go through this: Poker Positions Explained
Preflop
Total pot (Reward) = $1 (small blind) +$2 (big blind) + $2 (UTG’s call) + $5 (MP’s raise)
= $10
Amount to Call (Risk) = $5
Pot Odds = $10 (Reward):$5 (Risk)
= 2:1
You decide to call, and others fold. The total pot is now $15 ($10 in the pot + $5 your call)
Flop
On the flop, the MP makes a half-size pot bet of $7.5. What are the odds now?
Total Pot = $15 (already in the pot) + $7.5 (MP’s bet)
= $22.5
Amount to Call = $7.5
Pot Odds = $22.5:$7.5
= 3:1
You decide to call, and the total pot is now $30 ($22.5 in the pot +$7.5 your call).
Turn
On the turn, the MP makes a quarter of a pot-size bet of $7.5. What are the odds now?
Total Pot = $30 (already in the pot) + $7.5 (MP’s bet)
= $37.5
Amount to Call = $7.5
Pot Odds = $37.5:$7.5
= 5:1
You decide to call, and the total pot is now $45 ($37.5 in the pot + $7.5 your call).
River
On the river, the MP makes a full pot-size bet of $45. What are the odds now?
Total Pot = $45 (already in the pot) + $45 (MP’s bet)
= $90
Amount to Call = $45
Pot Odds = $90:$45
= 2:1
The pot odds on the river is now 2:1. So, if you call, you will get 2X the reward for your call.
As you can see, the odds increase or decrease on different streets. By using the poker odds, you get a clear picture of the reward you will earn for the risk you take.
A Shortcut to Know Your Pot Odds
An easy way to know the odds is to know how much is in the pot and the percentage of the pot your opponent bets.
- What’s the Pot Size?
- What Percentage of the Pot is Your Opponent Betting?
For example, if the pot is $100 and your opponent bets $25, you can say that he is making a 1/4 pot-size bet or he is betting 25% of the pot.
You can save this chart or print it and paste it on a wall next to your computer.
Why are Pot Odds used in Poker?
Pot odds tell you the ratio between the reward and the risk. It helps you to understand how much you need to risk to win the reward. But the real question is, “Is it worth the risk?” For that, you need to compare your card odds with the pot odds.
What are Card Odds?
It is the ratio of cards in the deck that you don’t want to the cards that you want. In other words, it is the ratio of unwanted cards and outs. If you don’t know what outs are, you can click here: How to Calculate Outs in Poker.
Card Odds = Cards we don’t need : Cards we need
Poker Odds Chart Explained Percentile
Let’s look at the example below to understand this concept better:
Your Hand: 7♦ 6♦
Flop: 8♦ K♣ 2♦
Your opponent bets $25 into a pot of $100. The total pot is $125. You need to call $25 for a chance to win $125.
So, the pot odds are $125:$25 or 5:1.
In this scenario, you have 9 outs to complete your flush draw. Let’s calculate the odds now.
- A deck has 52 cards.
- We know 5 cards out of those 52 cards – Our 2 Hole Cards and 3 cards on the Flop.
- 52 – 5 = 47. There are 47 cards in the deck that we don’t know.
- There are 9 outs that will help us to make the flush. We need those 9 cards (outs).
- 47 – 9 = 38. We don’t need those 38 cards.
- Card Odds = Cards we don’t need : Cards we need
- Card Odds = 38:9
- 38:9 can be simplified as 4.2:1 or roughly 4:1
The card odds tell us that the odds of hitting a diamond-suited card is 4:1. This means that there is a possibility that a diamond-suited card is in the deck once every 4 cards. In other words, for every 4 times, you won’t hit a diamond, 1 time you will.
Compare Pot Odds with Card Outs
The golden rule is that thepot odds should be greater than the card outs. When the odds from the pot are bigger than the odds of hitting an out, you will be winning more money than losing in the long run. So, the reward should be bigger than the risk.
Pot Odds > Card Outs
In the above example, we have the following odds:
Pot Odds = 5:1
Card Odds = 4:1
Pot Odds > Card Outs
We should call here as the pot odds are greater than card odds.
Card Odds Chart
It may be very difficult to do the addition and division to find the card odds while playing poker. You can save this card odds chart or print it to paste it on the wall. The chart has the common draw scenarios with the poker outs that you might face while playing poker. With a bit of practice, you will remember the important card odds.
* The last column All-in on the Flop (2 Cards to Come) is in a situation when you or your opponent go all-in on the flop. In an all-in situation, you don’t have to call bets on the turn or river. So, you will see 2 cards till the river. Seeing 2 cards instead of 1 improves your odds.
Let’s look at the example below for a quick exercise.
Your Hand: 7♦ 6♦
Turn: 8♦ K♣ 2♦ J♠
You have a flush draw with 9 outs.
Bet Size #1
Your opponent makes a quarter of a pot-size bet of $25 into a pot of $100. The total pot is now $125. You need to call $25 for a chance to win $125.
Pot Odds: 5:1 ($125:$25)
Card Odds: 4.1:1 (Look at the chart)
Pot Odds > Card Odds
Here, you can call as the pot odds are greater than the card odds. This means that you will be winning more money than losing in the long run.
Bet Size #2
In the same example, if your opponent makes a full pot-size bet of $100 into a pot of $100, the total pot becomes $200. You need to call $100 for a chance to win $200.
Pot Odds: 2:1 ($200:$100)
Card Odds: 4.1:1 (Look at the chart)
Card Odds > Pot Odds
Here, the card odds are greater than the pot odds. Although you might hit a flush on the river, calling here won’t be profitable in the long run. So, it will be wise to fold here.
Calculating Pot Odds Using the Percentage Method
Another way of calculating odds in poker is by using the percentage method. There are three simple steps in calculating the odds using the percentage method.
Step 1: Calculate the Final Pot
This is the major difference between the ratio method and the percentage method. In the percentage method, you need to add your call amount to the total pot to calculate the final pot. For example, if there is $6 in the pot and your opponent bets $3, the total pot becomes $9. Now add your call amount, which is $3, to calculate the final pot.
Total Pot = $6 (Already in the pot) + $3 (Opponent’s Bet)
= $9
Final Pot = $9 (Total Pot) + $3 (Call Amount)
= $12
Step 2: Divide the Call Amount by the Final Pot
Call Amount = $3
Final Pot = $12
3/12 = 0.25
Step 3: Multiply by 100
Multiply by 100 to get the percentage.
0.25 X 100 = 25%
So, the pot odds are 25%.
Poker Pot Odds Example Using the Percentage Method
Alert! This can be a bit mathematical but an easy hack to learn the odds quickly is available right after the example.
So, have some patience and continue reading…
Let’s review the example without looking at the hole cards to find the odds percentage.
You are on the Button in a $1/$2 6-max game. The Under the Gun (UTG) calls $2. The Middle Position (MP) raises to $5. Let’s calculate the odds.
Preflop
Total pot = $1 (small blind) +$2 (big blind) + $2 (UTG’s call) + $5 (MP’s raise)
= $10
Amount to Call = $5
Final Pot = $10 (Total Pot) + $5 (Call Amount)
= $15
Pot Odds = $5 (Call Amount) / $15 (Final Pot)
= 0.33
0.33 X 100 = 33%
So, the pot odds are 33%.
You decide to call, and others fold. The total pot is now $15 ($10 in the pot + $5 your call)
Flop
On the flop, the MP makes a half-size pot bet of $7.5. What are the odds now?
Total Pot = $15 (already in the pot) + $7.5 (MP’s bet)
= $22.5
Amount to Call = $7.5
Final Pot = $22.5 (Total Pot) + $7.5 (Call Amount)
= $30
Pot Odds = $7.5 (Call Amount) / $30 (Final Pot)
= 0.25
0.25 X 100 = 25%
So, the pot odds are 25%.
You decide to call, and the total pot is now $30 ($22.5 in the pot +$7.5 your call).
Turn
On the turn, the MP makes a quarter of a pot-size bet of $7.5. What are the odds now?
Total Pot = $30 (already in the pot) + $7.5 (MP’s bet)
= $37.5
Amount to Call = $7.5
Final Pot = $37.5 (Total Pot) + $7.5 (Call Amount)
= $45
Pot Odds = $7.5 (Call Amount) / $45 (Final Pot)
= 0.17
0.17 X 100 = 17%
So, the pot odds are 17%.
You decide to call, and the total pot is now $45 ($37.5 in the pot + $7.5 your call).
River
On the river, the MP makes a full pot-size bet of $45. What are the odds now?
Total Pot = $45 (already in the pot) + $45 (MP’s bet)
= $90
Amount to Call = $45
Final Pot = $90 (Total Pot) + $45 (Call Amount)
= $135
Pot Odds = $45 (Call Amount) / $135 (Final Pot) = 0.33
0.33 X 100 = 33%
So, the pot odds are 33%.
A Shortcut to Know Your Pot Odds Percentage
An easy way to know the odds is to know how much is in the pot and the percentage of the pot your opponent bets.
- What’s the Pot Size?
- What Percentage of the Pot is Your Opponent Betting?
For example, if the pot is $100 and your opponent bets $25, you can say that he is making a 1/4 pot-size bet or he is betting 25% of the pot.
You can save this pot odds chart or print it and paste it on a wall next to your computer.
How is the Percentage used in Poker?
In the percentage method, you need to find the card equity and compare it with odds percentage to make a decision. Card equity is as same as card odds. Instead of using a ratio, it is expressed in percentage.
How to Find Card Equity?
Card equity can be accurate if you know what range of hands your opponents are holding. There are many equity calculators available online. Since this is an introductory chapter on poker pot odds, we will teach you the simplest way to calculate card equity.
On the Flop Waiting for the Turn – Number of Outs X 4
On the Turn Waiting for the River – Number of Outs X 2
Let’s look at the example below to calculate the equity.
Your Hand: 7♦ 6♦
Flop: 8♦ K♣ 9♠
You have an open-ended straight draw with 8 outs.
Equity = 8 outs x 4
= 32%
Turn: 8♦ K♣ 9♠ 2♥
Equity = 8 x 2
= 16%
Compare Pot Odds with Equity
The golden rule is that theequity should be greater than the pot odds. When the equity is greater than the pot odds, you will be winning more money than losing in the long run. Here, the reward is bigger than the risk.
Equity > Pot Odds
Let’s look at the example below to compare the odds and equity.
Your Hand: 7♦ 6♦
Turn: 8♦ K♣ 2♦ J♠
You have a flush draw with 9 outs.
Bet Size #1
Your opponent makes a quarter of a pot-size bet of $25 into a pot of $100. The total pot is now $125. The final pot is $150 (total pot + call amount).
Pot Odds: 17% (25/150 X 100)
Equity: 9 (outs) X 2 (Turn)
= 18%
Equity > Pot Odds
Here, you can call as the equity is greater than the pot odds. This means that you will be winning more money than losing in the long run.
Bet Size #2

In the same example, if your opponent makes a full pot-size bet of $100 into a pot of $100, the total pot becomes $200. The final pot is $300 (total pot + call amount).
Pot Odds: 33% (100/300 X 100)
Equity: 9 (outs) X 2 (Turn)
= 18%
Pot Odds > Equity
Here, the pot odds are greater than the equity. Although you might hit a flush on the river, calling here won’t be profitable in the long run. So, it will be wise to fold here.
An easy way to find out how much equity is required for you to call is to find out the percentage of the pot your opponent bets. Remember these odds against your opponent’s bet sizes.
Conclusion
It might be a little overwhelming for the first time to grasp the concept of pot odds. However, with a bit of practice, you will get used to it. We recommend you to save all the charts or print them and paste it on the wall to take quick decisions. Poker is a mathematical game, and if you get your numbers right, you will always win money in the long run!
This post works with 5-card Poker hands drawn from a standard deck of 52 cards. The discussion is mostly mathematical, using the Poker hands to illustrate counting techniques and calculation of probabilities
Working with poker hands is an excellent way to illustrate the counting techniques covered previously in this blog – multiplication principle, permutation and combination (also covered here). There are 2,598,960 many possible 5-card Poker hands. Thus the probability of obtaining any one specific hand is 1 in 2,598,960 (roughly 1 in 2.6 million). The probability of obtaining a given type of hands (e.g. three of a kind) is the number of possible hands for that type over 2,598,960. Thus this is primarily a counting exercise.
___________________________________________________________________________
Preliminary Calculation
Usually the order in which the cards are dealt is not important (except in the case of stud poker). Thus the following three examples point to the same poker hand. The only difference is the order in which the cards are dealt.
These are the same hand. Order is not important.
The number of possible 5-card poker hands would then be the same as the number of 5-element subsets of 52 objects. The following is the total number of 5-card poker hands drawn from a standard deck of 52 cards.
The notation is called the binomial coefficient and is pronounced “n choose r”, which is identical to the number of -element subsets of a set with objects. Other notations for are , and . Many calculators have a function for . Of course the calculation can also be done by definition by first calculating factorials.
Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of “3 diamond, 2 heart” hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
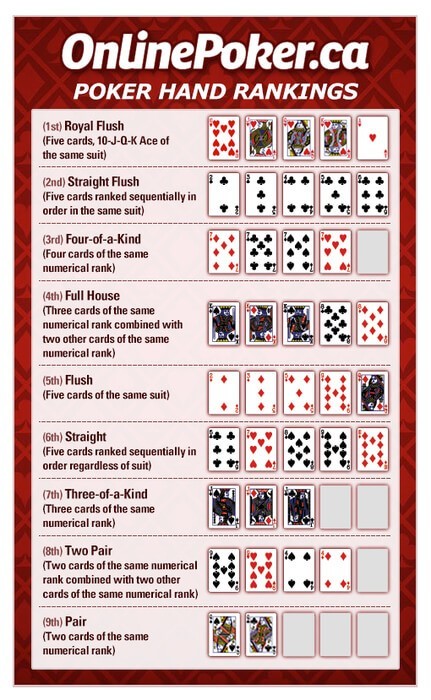
Here’s a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.
The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.
Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let’s fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2’s and choosing 2 cards out of the four 8’s. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.
High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Probabilities of Poker Hands
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
___________________________________________________________________________
2017 – Dan Ma